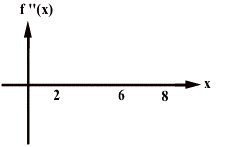Circle answers where appropriate.
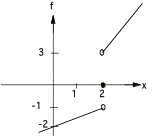
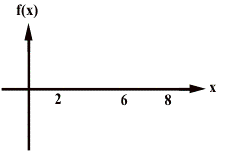
Use the function in the graph below for
problems 1 & 2:
1.
At which value(s) of x does the limit not exist ?
2.
Find 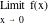 .
.
Use the piecewise continuous function
below for problems 3 - 5
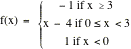 , Find:
, Find:
3. 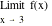
4.
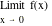
5.
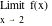
Use the Rules for Limits to evaluate:
If 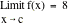 and
and 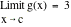 ,
,
6.
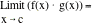
7.
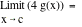
8.

Evaluate the limits.
9. 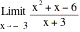
10. 
Find the Vertical Asymptote(s) for:
11. 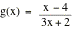
12.
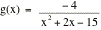
13.
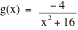
Find the Horizontal Asymptotes for :
14. 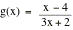
15.
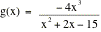
16.

17. If D represents the number of deer amd W represents the number of wolves
in the forest as a function of time, what sign would you expect dD/dW to have?
18.
Give the graphical definition of the derivative of y = f(x) at the point
(a, f(a)). You must use a complete sentences to get any credit at all.
19. Find the equation of the line tangent
to f(x) = 4x2 - 3x - 1 at x = 1.
20.
If the position of a rabbit is given by the function
x(t) = 2t2 - 4t +5, find her
speed and when t = 4 s.
What is her acceleration at this same
instant?
Differentiate the function:
(means find f ' (x) ).
21.
f(x) = 3sin x
22.
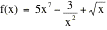
23.
f(x) = cos (¹)
24.
f(x) = 5cos (x)
25. f(x) = x2 + tan x
5 Points Bonus:
Does this function have a vertical
asymptote? If so, find it, if not,
explain why not.
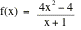
5
Points Bonus:
Draw the f(x) vs x and fÕÕ(x) vs x (assume the object starts at the origin
) graphs for the given fÕ(x) vs x graph.
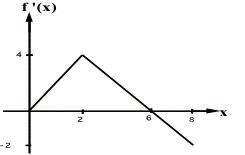

![]() .
.![]() , Find:
, Find:![]()
![]()
![]()
![]() and
and ![]() ,
, ![]()
![]()
![]()
![]()
![]()
![]()